- Home
- /
- Analytics
- /
- Forecasting
- /
- Re: ARIMA-transfer function
- RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- RSS Feed
- Permalink
- Report Inappropriate Content
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- RSS Feed
- Permalink
- Report Inappropriate Content
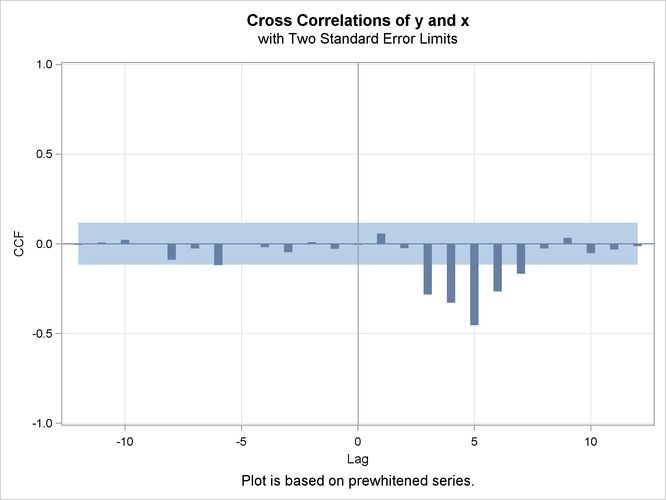
Begin your reference from lag 0.
There are three intervals at lags 0, 1, and 2 before the first significant spike ---> shift=3
A two spike build up at lags 4 and 5 following the first significant spike ---> numerator=1,2
After lag 5, there is decaying significance ---> denominator=1
If there was no decay (an immediate drop to non-significance after lag 5) ---> denominator=0
Your estimate statement in PROC ARIMA should look something like:
estimate input=(3$(1 2)/(1)X) method=ml; run;
Once fit, evaluate the residuals for appropriate AR and MA orders and re-estimate if necessary to obtain WN residuals.
- Mark as New
- Bookmark
- Subscribe
- Mute
- RSS Feed
- Permalink
- Report Inappropriate Content
Begin your reference from lag 0.
There are three intervals at lags 0, 1, and 2 before the first significant spike ---> shift=3
A two spike build up at lags 4 and 5 following the first significant spike ---> numerator=1,2
After lag 5, there is decaying significance ---> denominator=1
If there was no decay (an immediate drop to non-significance after lag 5) ---> denominator=0
Your estimate statement in PROC ARIMA should look something like:
estimate input=(3$(1 2)/(1)X) method=ml; run;
Once fit, evaluate the residuals for appropriate AR and MA orders and re-estimate if necessary to obtain WN residuals.
- Mark as New
- Bookmark
- Subscribe
- Mute
- RSS Feed
- Permalink
- Report Inappropriate Content
- Mark as New
- Bookmark
- Subscribe
- Mute
- RSS Feed
- Permalink
- Report Inappropriate Content
No problem, very glad to help.
You asked how to calculate the r-square of an ARIMA model. My first thought is why do you want to calculate r-square?
If you want a goodness-of-fit statistic to evaluate how well your model fits the data, I'd recommend choosing either AIC or SBC instead of r-square.
Unlike r-square that will never decrease as the number of model parameters increases, both AIC and SBC try to ensure the model is parsimonious. AIC and SBC are standard PROC ARIMA output when build a model.
That said, the link below walks through calculating r-square from output in PROC ARIMA.
http://support.sas.com/kb/37/955.html
- Mark as New
- Bookmark
- Subscribe
- Mute
- RSS Feed
- Permalink
- Report Inappropriate Content

The 2025 SAS Hackathon has begun!
It's finally time to hack! Remember to visit the SAS Hacker's Hub regularly for news and updates.
- Ask the Expert: Security Changes Affecting SAS Systems | 07-Oct-2025
- Fall 2025: MONSUG Meeting | 08-Oct-2025
- Ask the Expert: Wie integriere ich SAS Viya Inhalte in Microsoft 365? | 09-Oct-2025
- Ask the Expert: Creating PowerPoints With SAS – 2 | 16-Oct-2025
- Ask the Expert: Orchestrating Your Multi-Wave Campaigns Through Scheduled Journeys | 21-Oct-2025
- SAS Bowl LV, Agentic AI | 22-Oct-2025
- BASUG (Boston) SAS Blowout 2025 | 24-Oct-2025
