What is the significance behind confidence intervals and alpha value?

[Babloo]

In general modelling, we will reject the null hypothesis if P value is less than alpha value (P<0.05) and we fail to reject the null hypothesis if P value is greater than or equal (P>=0.05) to alpha value. Although I tried to understand the significance behind alpha value i could not understand it's significance.

Why we should reject the null hypothesis if P value is less than alpha value (P<0.05) for 95% CI? Can someone tell me the significance behind alpha value and confidence intervals?

Thanks!

[MichelleHomes]

The SAS/STAT user guide has some definitions that may help - SAS/STAT(R) 13.1 User's Guide - Hypothesis Testing, Power, Confidence Interval as well as this old SUGI paper - http://www2.sas.com/proceedings/sugi22/STATS/PAPER270.PDF

Kind Regards,

Michelle

[Babloo]

Thanks for the document.

However, I'm not clear on the point, 'Another common approach is to reject the null hypothesis when the %~o confidence intervals of the means do not overlap' from the documentation. If you can explain, it would be better for me to understand.

[Ksharp]

P value is actually the probability of  (H > H0 )   (H0 is  the estimator when H0 is true) .

And keep in your mind, all the estimators whether it is Normal estimator or Chi-square  estimator , they are all the estimator of deviation of expected value.

i.e. they all contains a   X-X0     ,it is a deviation , X0 is the estimator value when H0 is ture.

Take right test as an example:  If P(H > H0 ) = 0.001 , means there is very few estimator H greater than H0 ,which means H0 is very very large ,on account of benchmark of significant degree ALPHA=0.05 . therefore X-X0   is very very large , X is differently than X0 .

Xia Keshan

[Babloo]

What is H and X in your comments?

And keep in your mind, all the estimators whether it is Normal estimator or Chi-square  estimator , they are all the estimator of deviation of expected value.- Any possibilities to expand this statement? I'm still not clear.

[Ksharp]

Assuming we have a variable named X which conform to Normal Distribution .

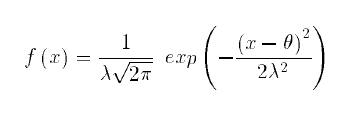
H is  hypothesis test  estimator which conform to Normal Distribution .

X is x in picture.

X0 is theta in picture . which is a value when H0 is true . e.x. H0: mu=0 , theta is 0 .

You see there is a measure of deviation ( x-theta ).

Chi-Square distribution also have such forum :  (x1- e)^2/e  +  (x2- e)^2/e   .....