- Home
- /
- Programming
- /
- Graphics
- /
- how to interpret my graph?
- RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
- Mark as New
- Bookmark
- Subscribe
- Mute
- RSS Feed
- Permalink
- Report Inappropriate Content
Hi,
I used PROC GLM for ANOVA.
ods html style=statistical; ods graphics on; title "Anova with Plots"; PROC GLM DATA = smoke3 order=data;; CLASS amt; MODEL fef=amt/solution; CONTRAST 'ns vs ps' amt 1 -1 0 0 0 0; CONTRAST 'ns vs ave inhale' amt 1 0 0 -.1 -.7 -.2; CONTRAST '# of smo' amt 0 0 0 -14 -4 18; CONTRAST 'ps vs ms' amt 0 -1 0 0 1 0; ESTIMATE 'ns vs ave inh' amt 1 0 0 -.1 -.7 -.2; ESTIMATE 'smo mn' intercept 70 amt 0 0 0 10 20 40 / divisor = 70; ESTIMATE 'nonsmo mn vs smo mn' amt 35 35 0 -10 -20 -40 / divisor = 70; ESTIMATE 'ps vs ms' intercept 70 amt 0 30 0 0 40 0 / divisor =70 ; lsmeans amt/pdiff adjust=tukey;; run; quit; ods graphics off; ods html close;
And i got following result:
Question: how to interpret my graph?
Thank you
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- RSS Feed
- Permalink
- Report Inappropriate Content
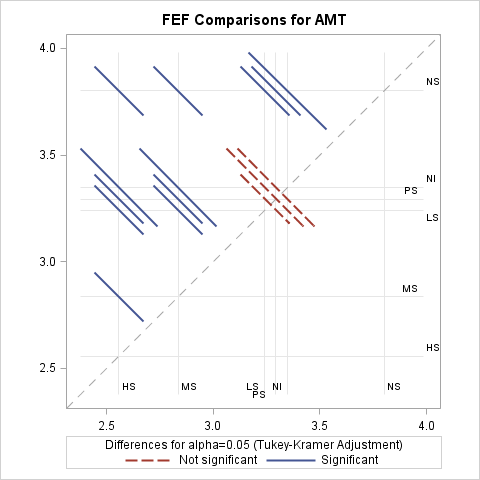
See p. 3 of "Interpreting the Differences Among LSMEANS in Generalized Linear Models" by Robin High.
Briefly, the blue lines represent pairs of groups whose mean differences are significantly different from zero. (That is, the means are sig diff from each other.) The red lines represent pairs that are NOT significantly different from zero. In your example, the mean difference between the NI and PS groups are not sig diff. Similarly, the mean difference between the NI-LS groups and the LS-PS groups are not sig different. The other pairwise comparison of means are significantly different from each other.
- Mark as New
- Bookmark
- Subscribe
- Mute
- RSS Feed
- Permalink
- Report Inappropriate Content
See p. 3 of "Interpreting the Differences Among LSMEANS in Generalized Linear Models" by Robin High.
Briefly, the blue lines represent pairs of groups whose mean differences are significantly different from zero. (That is, the means are sig diff from each other.) The red lines represent pairs that are NOT significantly different from zero. In your example, the mean difference between the NI and PS groups are not sig diff. Similarly, the mean difference between the NI-LS groups and the LS-PS groups are not sig different. The other pairwise comparison of means are significantly different from each other.

The 2025 SAS Hackathon has begun!
It's finally time to hack! Remember to visit the SAS Hacker's Hub regularly for news and updates.
Learn how use the CAT functions in SAS to join values from multiple variables into a single value.
Find more tutorials on the SAS Users YouTube channel.
SAS Training: Just a Click Away
Ready to level-up your skills? Choose your own adventure.